Problem 1 [35 points]
Consider a one dimensional Bravais lattice of lattice constant a . Let x label the position along this lattice, as shown below. Assume there is only a single ion at each BL site. If each ion contributes exactly one conduction electron, we learned in class that we expect the lowest band will be half filled and the system will behave like a metal. However, in the 1930's, Rudolf Peierls showed how such a situation can result in an insulator! This effect is now known as the Peierls instability. The purpose of this problem is to have you figure out the mechanism for this effect.

a) What is the primitive vector for this Bravais lattice? What range of x is the Wigner-Seitz cell? What are the vectors of the reciprocal lattice? What range of k is the 1st Brillouin Zone?
b) The ions of the lattice give rise to a periodic potential U(x). Assuming the effect of this potential can be treated within the weak potential approximation. Sketch the band structure in the reduce zone scheme, showing the lowest two bands. If each ion of the lattice contributes m conduction electrons, for which values of m will the system be a metal? an insulator? Explain your reasoning.
c) Imagine that the ions of the lattice undergo a distortion as shown below (every other ion moves ot the left an amount δx). How would you now describe the below crystal structure? What is the primitive vector? What range of x is the Wigner-Seitz cell? What are the vectors of the reciprocal lattice? What range of k is the 1st Brillouin Zone?
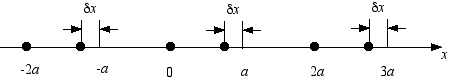
d) Sketch the band structure in the reduced zone scheme for this deformed lattice. Show enough bands that your sketch shows the same number of electron states as represented in your sketch of part (b) for the undistorted lattice. If each ion contributes exactly one conduction electron, will the deformed lattice be a metal or an insulator?
e) Assume that the total ion potential U(x) can be written as a sum over the individual potentials from each ion, i.e. U(x) = ∑i V(x - xi), where the xi give the positions of the ions, and V(x) is the potential of a single ion centered at x = 0. If one writes U(x) = Uo(x) + δU(x), where Uo(x) is the potential of the undistorded lattice and δU(x) is the correction due to the distortion, what is the period of Uo(x)? of δU(x)? Write an explicit expression for δU(x) in terms of V(x), assuming the distortion δx is small. How does δU(x) vary with δx?
f) Give a crude estimate of how the total electronic energy density (energy per length) changes due to the lattice distortion. Does it increase or decrease? (Assume one conduction electron per ion as in part (d).) Hint: you should do this by making a physically reasonable estimate, rather than an exact analytical calculation (although the exact calculation can be done and gives an important correction to the naive estimate).
g) Assume we can model the elastic energy on the ion-ion interaction as a set of springs connecting nearest neighbor ions, i.e. the elastic energy of a pair of ions is (1/2)C(xi+1 - xi - a)2, where C is the spring constant. What is the elastic energy density (energy per length) due to the distortion? Assume that the total energy of the sytem is the sum of the elastic and electronic energies. Does the lattice prefer to make the distortion or not?
Problem 2 [20 points]
Consider a two dimensional square Bravais lattice with lattice constant a. Suppose the periodic ionic potential is given by,
U(x, y) = -4Ucos(2πx/a)cos(2πy/a)
a) Find the Fourier components UK for reciprocal lattice vectors K.
b) For k = (k, 0) along the kx axis, how does the single electron energy ε(k) behave at the boundary of the first Brillouin Zone, (π/a, 0)?
c) For k = (k, k) along the diagonal of the first Brillouin Zone, how does ε(k) behave at the corner of the first Brillouin Zone, (π/a, π/a)?
