|
|

Home
Contact
Info
Course
Info
Calendar
Homework
Lecture
Notes
|
|
|
|
Physics 415: Electromagnetic Theory I
Prof. S. Teitel stte@pas.rochester.edu ----- Fall 2003
Problem Set 3
Due Wednesday, October 15, in lecture
- Problem 1 [10 points total]
Consider an infinitely long grounded metal cylinder, of radius R, placed at right angles to an otherwise uniform electric field Eo.
a) Find the potential outside the cylinder.
b) Find the surface charge induced on the cylinder.
- Problem 2 [10 points total]
A spherical shell of radius R carries a uniform surface charge  o on the northern hemisphere, 0< o on the northern hemisphere, 0< < < /2, and an equal but opposite uniform surface charge
- /2, and an equal but opposite uniform surface charge
- o on the southern hemisphere, o on the southern hemisphere,
 /2< /2< < < . Calculating the coefficients in the Legendre expansion up to l=6, . Calculating the coefficients in the Legendre expansion up to l=6,
a) Find the potential outside the sphere.
b) Find the potential inside the sphere.
- Problem 3 [20 points total!]
a) Consider an infinitesmally thin charged disk of radius R and uniform surface charge density  o, that is lying in the xy plane centered on the origin. Using the Coulomb formula for the potential, o, that is lying in the xy plane centered on the origin. Using the Coulomb formula for the potential,
do the integral to find the exact value of the potential 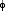 for points along the z axis, both above and below the disk (make sure you get this part correct before proceeding with the following parts!). [5 pts] for points along the z axis, both above and below the disk (make sure you get this part correct before proceeding with the following parts!). [5 pts]
b) Since the above problem of the charged disk has azimuthal symmetry, we can express the solution for the potential 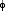 (r) in terms of a Legendre polynomial series using the method of separation of variables. In this case, the "boundary condition" that determines the unknown coefficients of this series is the requirement that the potential agree with the known exact values along the z axis, as computed in part (a). Find the coefficients of this expansion up to order l=5, for |r|>R and |r|<R, both above and below the disk. (Jackson section 3.3 might be helpful) [10 pts] (r) in terms of a Legendre polynomial series using the method of separation of variables. In this case, the "boundary condition" that determines the unknown coefficients of this series is the requirement that the potential agree with the known exact values along the z axis, as computed in part (a). Find the coefficients of this expansion up to order l=5, for |r|>R and |r|<R, both above and below the disk. (Jackson section 3.3 might be helpful) [10 pts]
c) Using your results of part (b), compute the electric field E just above and just below the disk, and explicitly show that the discontinuity in E is given by the surface charge density  o, as we know from general principles that it must be. [5 pts] o, as we know from general principles that it must be. [5 pts]
|

