|
|

Home
Contact
Info
Course
Info
Calendar
Homework
Lecture
Notes
|
|
|
|
Physics 415: Electromagnetic Theory I
Prof. S. Teitel stte@pas.rochester.edu ----- Fall 2004
Problem Set 1
Due Wednesday, September 29, in lecture
- Problem 1 [10 points]
Using Dirac delta functions in the appropriate coordinate system, express the following charge distributions as three dimensional charge densities  (x). (x).
a) In spherical coordinates, a charge Q uniformly distributed over a spherical shell of radius R.
b) In cylindrical coordinates, a charge  per unit length uniformly distributed over a cylindrical surface of radius b. per unit length uniformly distributed over a cylindrical surface of radius b.
c) In cylindrical coordinates, a charge Q spread uniformly over a flat circular disc of negligible thickness and radius R.
d) The same as (c), but using spherical coordinates.
- Problem 2 [10 points]
Suppose the electric and magnetic fields, E and B, are given by scalar and vector potentials, 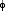 and A, that are not in the Lorentz gauge. Show that one can always find a gauge transformation that takes one to new potentials which are in the Lorentz gauge. Specifically, if and A, that are not in the Lorentz gauge. Show that one can always find a gauge transformation that takes one to new potentials which are in the Lorentz gauge. Specifically, if  is the scalar function of the gauge transformation, find an equation to solve for is the scalar function of the gauge transformation, find an equation to solve for  in terms of the orignial in terms of the orignial 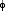 and A, such that the new and A, such that the new 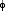 ´ and A´ are in the Lorentz gauge. ´ and A´ are in the Lorentz gauge.
- Problem 3 [20 points]
In lecture we discussed the decomposition of any vector function
f(r) into its curlfree and divergenceless parts,
f||(r) and f (r), as given by
Helmholtz's theorem: (r), as given by
Helmholtz's theorem:
f(r) = f||(r) + f  (r) (r)
where
f||(r) = — 
|
[
|
1
4
|
|
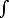
|
d3r´ |
 ´ · f(r´) ´ · f(r´)
|
r-r´|
|
|
]
|
and
f (r) = (r) =  
|
[
|
1
4
|
|
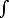
|
d3r´ |
 ´ ´  f(r´) f(r´)
|
r-r´|
|
|
]
|
Consider f(k), the Fourier transform of f(r):
|
f(r) =
|
1
(2 )3 )3
|
+
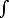
-
|
d3k eik · r
f(k) |
Using the results above, find expressions for the Fourier transforms of the curlfree (longitudinal) and divergenceless (transverse) parts of f(r).
It may help to know that the Fourier transform of
1/|r|
is
4 /k2, and that the Fourier transform of the Dirac delta function /k2, and that the Fourier transform of the Dirac delta function  (r) is eik · r (r) is eik · r
- Problem 4 [10 points]
Prove this mean value theorem: For charge-free space, the value of the electrostatic potential at any point in space is equal to the average of the potential over the surface of any sphere centered on that point.
Hint: Use the fact that where there are no charges,  2 2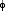 =0. Functions which satisfy this Laplace's equation are called harmonic functions; harmonic funtions obey the above mean value theorem. =0. Functions which satisfy this Laplace's equation are called harmonic functions; harmonic funtions obey the above mean value theorem.
|

