Problem Set 4

Due Wednesday, October 20, in lecture

Problem 1 [15 points]
Consider a line charge density (z) that is localized on the z axis from z=-a to z=+a. By considering the monopole, dipole, and quadrapole moments of the charge distribution, find an approximation for the potential 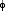(r) to leading order only in the multipole expansion, for each of the following three cases:
a) (z) = _ocos(z/2a)
b) (z) = _osin(z/a)
c) (z) = _ocos(z/a)
Problem 2 [15 points]
Consider a uniformly charged disk of radius R in the xy plane at z=0, centered at the origin. Find the monopole moment, the dipole moment vector, and the quadrapole moment tensor. Use these moments to write an approximation for the potential 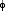(r) for r far from the disk. Compare your results with what you found in problem 3b of Problem Set 3.
Problem 3 [15 points]
a) Consider a spherical shell of radius R, with uniform surface charge density _o, centered on the origin. The shell is spining counterclockwise about the z axis with angular velocity . Find the magnetic vector potential A(r), far from the sphere, using the magnetic dipole approximation. Find the magnetic field B within this approximation.
b) Using the method of separation of variables, as applied to the scalar magnetic potential 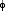_M, find an expression for the exact magnetic field B both inside and outside the spining charged shell of part a. How does your answer for the field outside compare with that obtained by the magnetic dipole approximation in part a?

Last update: Tuesday, August 21, 2007 at 11:05:17 PM.