- Problem 1 [10 points]
Prove this mean value theorem: For charge-free space in the electrostatic limit, the value of the electrostatic potential φ at any point in space is equal to the average of the potential over the surface of any sphere centered on that point.
Hint: Use the fact that where there are no charges ∇2φ=0. Functions which satisfy this Laplace's equation are called harmonic functions; harmonic functions obey the above mean value theorem.
- Problem 2 [10 points]
a) In lecture we solved the problem of the electric field from a spherical shell of radius R with uniform surface charge density σ=q/(4πR2). Consider now the problem where this shell is of finite thickness d. That is, there is a uniform charge density ρ in a spherical shell of finite thickness from radius R to radius R+d, such that the total charge on this shell is q. Find the potential φ(r) by solving Poisson's equation (there may be easier ways to do it, but do it this way!), then take the gradient to get E(r). Sketch φ(r) and E(r) vs r. Now take the limit d→0 keeping ρd=σ constant. Compare your result with the case of the infinitesmally thin shell done in lecture.
b) Consider an infinitesmally thin spherical shell of radius R with a total charge q uniformly distributed over its surface, and a concentric infinitesmally thin spherical shell of radius R+d with total charge -q uniformly distributed over its surface. Find the potential φ(r) by solving Poisson's equation for this geometry, then take the gradient to get E(r). Sketch φ(r) and E(r) vs r. Now take the limit d→0 keeping qd constant. What do you find? This is the limit of an infinitesmally thin dipole layer.
- Problem 3 [10 points]
Consider a grounded, conducting, spherical shell of outer radius b and inner radius a.
Using the method of images, dicuss the problem of a point charge q inside the shell, i.e. at a distance r<a from the center. Find
a) the potential inside the sphere;
b) the induced surface charge density on the inner surface of the shell at r=a; what is the total induced charge?
c) the magnitude and direction of the force acting on q. Does q get pushed towards the center, or away from the center?
d) Is there any change in the solution if the sphere is kept at a fixed potential φo? If the sphere has a fixed total charge Q?
- Problem 4 [5 points]
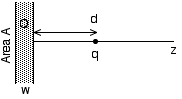
Consider a point charge q a distance d in front of a plane conducting slab of thickness w, as shown below. The slab has a fixed net charge Q on it. Find the electric field on both sides of the slab. Find the force between the charge q and the slab. For this problem, assume that the side area of the slab A is finite, so that the average surface charge Q/2A is finite; however you make work the problem out ignoring edge effects, i.e. assuming the plane is effectively infinite.
- Problem 5 [10 points]
A line charge λ is placed parallel to, and a distance R away from, the axis of a conducting cylinder of radius b that is held at fixed voltage such that the potential vanishes at infinity. Find
a) the magnitude and position of the image charge(s)
b) the potential at any point (expressed in polar coordinates with the origin at the axis of the cylinder and the direction from the origin to the line charge as the x axis)
c) the induced surface charge density, and plot it as a function of angle for R/b = 2 and 4 in units of λ/2πb
d) the force on the line charge per unit length