|
|

Home
Contact
Info
Course
Info
Calendar
Homework
Lecture
Notes
|
|
|
|
Physics 418: Statistical Mechanics I
Prof. S. Teitel stte@pas.rochester.edu ----- Spring 2006
Problem Set 6
Due Tuesday, April 25, in lecture
- Problem 1 [15 points]
Consider a degenerate Fermi gas of non-interacting, non-relativisitic, particles in two dimensions (this might be a model for electrons in a thin metallic film).
a) Find the density of states g(ε).
b) Find the Fermi energy and the T=0 energy density.
c) Using the the fact that the particle density n is given by
n = 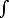 dε dε |
g(ε)
eβ(ε-µ)+1 |
find the chemical potential as a function of temperature, µ(T), for fixed density n, by doing this integral exactly.
You may have to look up an integral in an integral handbook! Using the exact expression for µ(T), find a simpler approximation that holds at low T<<TF. Does µ(T) have a power series expansion in T at low T?
- Problem 2 [15 points]
N Fermions A of spin 1/2 are introduced into a large volume V at
temperature T. Two Fermions may combine to create a Boson with
spin 0 via the interaction,
A + A <--> A2
Creation of the molecule A2 costs energy εo > 0.
At equilibrium, the system will contain NF Fermions and
NB Bosons. Provide expressions from which the ratio
NB/NF can be calculated, and perform the
calculation explicitly for T=0. What would this (T=0) ratio be,
if the particles were classical (i.e. quantum statistics can be neglected).
Explain the difference.
- Problem 3 [15 points]
Consider an ideal Bose gas composed of molecules with an internal
degree of freedom. Assume that this internal degree of freedom
can have only one of two energy values, the ground state
εo = 0, and an excited state, ε1 > 0.
Determine the Bose Einstein condensation temperature of the gas
as a function of ε1. Show in particular that for
ε1/kBT >> 1,
Tc
Tco |
= 1- |
2e
-ε1/kBT
3ζ(3/2) |
where Tco is the transition temperature when ε1
is infinite, and ζ is the Riemann zeta function.
|

