|
|

Home
Contact
Info
Course
Info
Calendar
Homework
Lecture
Notes
|
|
|
|
Physics 418: Statistical Mechanics I
Prof. S. Teitel stte@pas.rochester.edu ----- Spring 2006
Problem Set 7
Due Wednesday, May 3, 5 pm in my mailbox outside department office
- Problem 1 [15 points]
In this problem we show how the Ising model can also be used as a model for the liquid to gas phase transition. The model outlined below is known as the lattice gas model.
Consider a classical gas of indistinguishable point particles with a pairwise interaction u(r) between them. The canonical partition function for No such particles is:
|
QNo(T,V) =
|
1
No! h3No |
(
|
No
Π
i=1 |
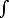 d3pi d3pi
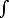 d3ri
) d3ri
)
|
e-β[Σipi2/2m + Σi,ju(ri-rj)]
|
As for non-interacting particles, one can easily do the momentum integrations to get:
|
QNo(T, V) =
|
1
λ3No |
CNo(T, V)
|
where λ is the usual thermal wavelength of the particles, and,
| CNo(T, V) =
|
1
No!
|
(
|
No
Π
i=1 |
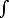 d3ri
) d3ri
)
|
e-βΣi,ju(ri-rj)
|
is the configuration space integral.
Consider now approximating this configuration space integral by the following lattice model. Replace the continuous space of volume V, with a discrete cubic lattice of N grid points. The lattice spacing of this grid is ao so that V=Nao3. Label the discrete sites of this grid by the index i (so now i labels grid sites rather than particles), and define a variable ni on each site such that ni can take only two values, 0 or 1. When ni=0, the grid site i is empty. When ni=1, the grid site is occupied by a particle. Particles interact with each other only when they occupy nearest neighboring sites of the grid, in which case they have an attractive interaction energy -K. We can think of this approximation as modeling an interaction potential u(r) that has a hard core repulsion for |r|<ao (so that no two particles occupy the same site, i.e. ni is never bigger than 1), has an attractive interaction for ao<|r|<2ao, and then no interaction for greater separations. The Hamiltonian for this lattice model is then,
where the sum is over nearest neighboring bonds of the grid. The configuration integral is then approximated by summing
| CNo(T, V) = ao3No |
Σ
{ni}
|
e-βH[ni]
|
over all possible configurations of the site occupation variables {ni}, subject to the constraint that No = Σi ni.
a) Map the above lattice gas model onto an Ising model, i.e. find a relation between the parameters of the lattice gas, and the parameters J and h of the Ising model. (Hint: it is important to remember the contribution from the momentum integrations to do this properly - this is a point that is missed in many textbooks!). In what ensemble (constant magnetization? constant magnetic field?) is this corresponding Ising model?
b) Now tranform from the canonical to the grand canonical ensemble,
|
L(T,V,µ) =
|
∞
Σ
No=0
|
zNoQNo(T, V)
|
where z = eβµ is the fugacity. Now what is the mapping from this grand canonical L to the Ising model. In what ensemble is the Ising model now? Based on your knowledge of the behavior of the Ising model, make a sketch of the phase diagram for the lattice gas model in the T-µ plane, showing the first order coexistence line, and the 2nd order critical end point.
c) Find expressions for the lattice gas density of particles, the lattice gas pressure, and the lattice gas isothermal compressibility, in terms of corresponding quantities for the Ising model, using the grand canonical ensemble of part (b).
- Problem 2 [15 points]
Consider the Ising model. The magnitization density is m, and the magnetic field is h. The magnetic susceptibility is then defined by
where the derivative is evaluated at h = 0.
Derive an expression for χ in terms of the fluctuations in magnetization m.
(Hint: recall, we had a similar expression for specific heat in terms of fluctuations in energy.)
Show from this result that fluctuations in magnetization become negligible in the thermodynamic limit, except at a critical point.
- Problem 3 [15 points]
Consider a Landau theory for the liquid-gas phase transition. This transition is characterized by a 1st order coexistence curve in the pressure-temperature plane, denoted p*(T), that ends in a 2nd order critical end point at temperature Tc and pressure pc = p*(Tc). We can imagine smoothly extending the curve p*(T) so that it is defined even for T > Tc. The particle density, n(T, p), depends on temperature and pressure, and along the coexistance curve can take two possible values, n(T, p*(T)) = ngas(T) or nliq(T). The difference,
nliq(T) - ngas(T), vanishes as T→Tc.
If nc = n(Tc, pc) is the density at the critical point, we can expand the free energy density about nc as a Taylor series in δn ≡ n - nc,
f(δn) = fo + c1(δn) + c2(δn)2 + c3(δn)3 + c4(δn)4 + O((δn)5)
Unlike the case of the Ising model, there is no symmetry principle that automatically rules out the odd powered terms in the expansion. The coeficients
c1, c2, c3, c4 are some as yet unspecified functions of p and T. We will ignore all terms higher than (δn)4.
a) Show that by transforming to a new variable
φ ≡ δn - δno
with δno a suitably chosen constant that depends on the coeficients ci, that the free energy can be rewritten in the form
f(φ) = fo - hφ + aφ2 + bφ4
The above form is exactly that of the Ising model, where φ is the order parameter,
and h the ordering field.
b) What is the location of the coexistence curve, and the critical point, in terms of the parameters of f(φ)? What is the location of the coexistence curve and the critical point in terms of the original coeficients c1, c2, c3, and c4? How do nliq and ngas behave as T→Tc? What is the physical meaning of the quantity δno?
|

