To speak with General Relativity one need to define some of the symbol used.
is the metric on the manifold. It is used
to define distances. From it we define the
Christoffel symbol [glo]Christoffel symbol
[glo]
There is another formula for this symbol, the one from the
difference between the derivative and the covariant
derivative, namely 
.
The Riemann tensor [glo]Riemann tensor
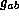[glo]
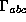is defined as the value of
the non-commutation of the covariant derivative
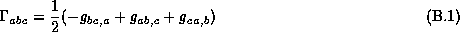. For computation there is also a formula
with the second derivative of the metric (through the
Christoffel symbol).
From the Riemann tensor the Ricci tensor [glo]Ricci tensor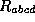 
[glo]
is defined by contraction.
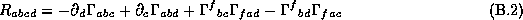
The Ricci curvature [glo]Ricci curvature R [glo]R.
Finally the Einstein equation [glo]Einstein equation is
where we have the energy-momentum tensor [glo]
[glo]energy-momentum tensor 
. It represents the distribution of matter in the universe.
