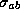 everywhere if it is zero at one point and
everywhere if it is zero at one point and 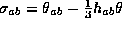 (this come from the geodesic equation).
(this come from the geodesic equation).
By going through the demonstration of the Raychaudhuri equation one can notice that for the case of geodesics we have 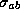 everywhere if it is zero at one point and
everywhere if it is zero at one point and 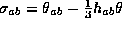 (this come from the geodesic equation).
(this come from the geodesic equation).
It can be interesting to see what it means in Fluids Dynamics.
As 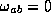 is the non-linear term, this say that a geodesic behave linearly.
is the non-linear term, this say that a geodesic behave linearly.
 gives us
gives us 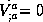 , it is symmetric. Then
, it is symmetric. Then 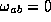 . So this show that geodesics can be associated with the flow line.
. So this show that geodesics can be associated with the flow line.
If our interest is about the derivative of  then it is obvious that we are interested in a compressible flow (
then it is obvious that we are interested in a compressible flow (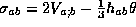 ).
).
Less obvious is the fact that we are interested in a non-isentropic flow (if isentropic then the shear tensor is reduce to the pressure term).