Problem 1 [15 points total]
In lecture we saw that the quantum N particle canonical
partition function could be written as a series involving
0-particle, 2-particle, 3-particle, ...,and N-particle exchanges.
The first two terms in this series were,
| QN(T,V) = |
(VN/
N!  3N) |
[ 1 ± V -2 |
i<j |
 |
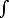d3ri |
|
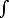d3rj |
f(ri-rj)
f(rj-ri) |
] |
where
f(r)=e-
r2/2 and is the thermal wavelength. The (+) sign is for bosons, and the (-) sign is for fermions.
The first term above gives the classical partition function, while
the second term can be viewed as the leading quantum correction
(in the limit that quantum corrections are small).
For the calculations below,
you may assume that this quantum correction term is small.
a) Explicitly evaluate the integrals to compute the above partition function. [5 pts]
b) Find the corresponding Helmholtz free energy. [5 pts]
c) Using your result in part (b), find the corresponding equation of state. How does the leading quantum
correction change the usual ideal gas law, pV = NkBT? [5 pts]
= 
.
)
where